Pengguna:Dedhert.Jr/Uji halaman 17: Perbedaan antara revisi

← Revisi sebelumnya Revisi selanjutnya →

Konten dihapus Konten ditambahkan

Sebaris

Revisi per 8 Februari 2022 13.37

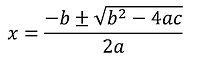
Rumus kuadrat atau dikenal sebagai rumus ABC.

Dalam aljabar elementer, rumus kuadrat adalah sebuah rumus yang mencari sebuah variabel yang tidak diketahui pada persamaan kuadrat.^[1] Terkadang, rumus ini disebut juga sebagai rumus ABC karena terkandung tiga variabel pada persamaan tersebut, yakni $a$ , $b$ , dan $c$ ,^[2] atau rumus kecap, yang merujuk ke salah satu kecap terkenal di Indonesia, yaitu kecap ABC.^[3]

Ringkasan

Bentuk umum persamaan kuadrat dapat ditulis sebagai

ax^{2}+bx+c=0

,

dimana $x$ adalah variabel yang tidak diketahui, dan $a,b,c$ adalah koefisien real (dengan $a\neq 0$ ), memberikan solusi $x$ :

x={\frac {-b\pm {\sqrt {b^{2}-4ac}}}{2a}}

.

Karena adanya tanda plus dan minus, rumus tersebut mempunyai dua solusi, yaitu:

x={\frac {-b+{\sqrt {b^{2}-4ac}}}{2a}}

dan

x={\frac {-b-{\sqrt {b^{2}-4ac}}}{2a}}

.

Penurunan

Berbagai bukti dan penurunan terhadap rumus kuadrat dapat dilakukan dengan berbagai cara, seperti menyelesaikan dalam bentuk kuadrat sempurna, substitusi, identitas aljabar, dan Lagrange resolvent.

Bentuk kuadrat sempurna

Metode singkat

Adapun metode singkat bentuk kuadrat sempurna:

Multiply each side by $4a$ ,
Rearrange.
Add $b^{2}$ to both sides to complete the square.
Take the square root of both sides.
Isolate $x$ .

In which case, the quadratic formula can also be derived as follows:

{\begin{aligned}ax^{2}+bx+c&=0\\4a^{2}x^{2}+4abx+4ac&=0\\4a^{2}x^{2}+4abx&=-4ac\\4a^{2}x^{2}+4abx+b^{2}&=b^{2}-4ac\\(2ax+b)^{2}&=b^{2}-4ac\\2ax+b&=\pm {\sqrt {b^{2}-4ac}}\\2ax&=-b\pm {\sqrt {b^{2}-4ac}}\\x&={\frac {-b\pm {\sqrt {b^{2}-4ac}}}{2a}}\ \ .\end{aligned}}

Diberikan persamaan $ax^{2}+bx+c=0$ , dengan membagi kedua ruas dengan $a$ akan memperoleh $x^{2}+{\frac {b}{a}}x+{\frac {c}{a}}=0$ . Selanjutnya kurangi kedua ruas dengan ${\textstyle -{\frac {c}{a}}}$ .

x^{2}+{\frac {b}{a}}x=-{\frac {c}{a}}

.

Pada ruas kiri, kita dapat mengubahnya menjadi bentuk kuadrat dengan menambahkan ${\textstyle \left({\frac {b}{2a}}\right)^{2}}$ pada kedua ruas.

\left(x+{\frac {b}{2a}}\right)^{2}=-{\frac {c}{a}}+{\frac {b^{2}}{4a^{2}}}

.

Pada ruas kanan, penyebutnya disamakan. Kedua ruas diakarkuadratkan dan dikurangi dengan ${\textstyle -{\frac {b}{a}}}$ sehingga kita memperoleh rumus kuadrat.

Melalui teknik yang serupa, terdapat cara sederhana dalam menurunkan rumus ini.^[4] This derivation of the quadratic formula is ancient and was known in India at least as far back as 1025.^[5] Compared with the derivation in standard usage, this alternate derivation avoids fractions and squared fractions until the last step and hence does not require a rearrangement after step 3 to obtain a common denominator in the right side.^[4]

Substitusi

Identitas aljabar

Lagrange resolvent

Rujukan

^ M.Pd, Sri Jumini, S. Pd (2017-11-20). Buku Ajar Matematika Dasar Untuk Perguruan Tinggi. Penerbit Mangku Bumi. hlm. 36. ISBN 978-602-52256-2-8.
^ "Rumus ABC - Pengertian, Soal, Pembahasan | dosenpintar.com". dosenpintar.com. Diakses tanggal 2022-02-07.
^ Mauhibah, Al Jupri, Rohma (2014-01-01). Trik Cerdas Paling Cadas Pintar Matematika SMA. PandaMedia. hlm. 64. ISBN 978-979-780-658-3.
^ ^a ^b Hoehn, Larry (1975). "A More Elegant Method of Deriving the Quadratic Formula". The Mathematics Teacher. 68 (5): 442–443. doi:10.5951/MT.68.5.0442.
^ Smith, David E. (1958). History of Mathematics, Vol. II. Dover Publications. hlm. 446. ISBN 0486204308.

Daftar pustaka

Bentuk kuadrat sempurna

CCSS HSA-REI.B.4 Completing the Square to Solve Quadratic Equations: Aligns to CCSS HSA-REI.B.4: Solve quadratic equations in one variable. (dalam bahasa Inggris). Lorenz Educational Press. 2014-01-01. hlm. 51. ISBN 978-0-7877-1056-9.
Hanna, Gila; Jahnke, Hans Niels; Pulte, Helmut (2009-12-04). Explanation and Proof in Mathematics: Philosophical and Educational Perspectives (dalam bahasa Inggris). Springer Science & Business Media. hlm. 91. ISBN 978-1-4419-0576-5.

Varberg, Dale. Kalkulus, Edisi Kesembilan, Jilid 1. Penerbit Erlangga. hlm. 14. ISBN 0-13-1429-24-8.

[1] M.Pd, Sri Jumini, S. Pd (2017-11-20). Buku Ajar Matematika Dasar Untuk Perguruan Tinggi. Penerbit Mangku Bumi. hlm. 36. ISBN 978-602-52256-2-8.

[2] "Rumus ABC - Pengertian, Soal, Pembahasan | dosenpintar.com". dosenpintar.com. Diakses tanggal 2022-02-07.

[3] Mauhibah, Al Jupri, Rohma (2014-01-01). Trik Cerdas Paling Cadas Pintar Matematika SMA. PandaMedia. hlm. 64. ISBN 978-979-780-658-3.

[Hoehn1975-4] Hoehn, Larry (1975). "A More Elegant Method of Deriving the Quadratic Formula". The Mathematics Teacher. 68 (5): 442–443. doi:10.5951/MT.68.5.0442.

[Smith1958-5] Smith, David E. (1958). History of Mathematics, Vol. II. Dover Publications. hlm. 446. ISBN 0486204308.

[1]

[2]

[3]

[4]

[5]

@@ Baris 23: / Baris 23: @@
 ==== Bentuk kuadrat sempurna ====
 {{Sidebox|above='''Metode singkat'''
-Adapun metode singkat bentuk kuadrat sempurna:}}
+|below=Adapun metode singkat bentuk kuadrat sempurna:
-Diberikan persamaan <math>ax^2 + bx + c = 0</math>, dengan membagi kedua ruas dengan <math>a</math> akan memperoleh <math>x^2 + \frac{b}{a}x + \frac{c}{a} = 0</math>. Selanjutnya kurangi kedua ruas dengan <math display="inline">-\frac{c}{a}</math>.
-: <math>x^2 + \frac{b}{a}x = -\frac{c}{a}</math>.
-Pada ruas kiri, kita dapat mengubahnya menjadi bentuk kuadrat dengan menambahkan <math display="inline">\left(\frac{b}{2a}\right)^2</math> pada kedua ruas.
-: <math>\left(x + \frac{b}{2a}\right)^2 = -\frac{c}{a} + \frac{b^2}{4a^2} </math>.
-Pada ruas kanan, penyebutnya disamakan. Kedua ruas diakarkuadratkan dan dikurangi dengan <math display="inline">-\frac{b}{a}</math> sehingga kita memperoleh rumus kuadrat.
-Completing the square can also be accomplished by a sometimes shorter and simpler sequence:<ref name="Hoehn1975">{{cite journal|last=Hoehn|first=Larry|year=1975|title=A More Elegant Method of Deriving the Quadratic Formula|journal=The Mathematics Teacher|volume=68|issue=5|page=442&ndash;443|doi=10.5951/MT.68.5.0442}}</ref>
 # Multiply each side by ''<math>4a</math>'',
 # Rearrange.
@@ Baris 53: / Baris 41: @@
 ax &= -b \pm \sqrt{b^2-4ac} \\
 x &= \frac{-b\pm\sqrt{b^2-4ac }}{2a}\ \ .
-\end{align}</math>
+\end{align}</math>}}
+Diberikan persamaan <math>ax^2 + bx + c = 0</math>, dengan membagi kedua ruas dengan <math>a</math> akan memperoleh <math>x^2 + \frac{b}{a}x + \frac{c}{a} = 0</math>. Selanjutnya kurangi kedua ruas dengan <math display="inline">-\frac{c}{a}</math>.
+: <math>x^2 + \frac{b}{a}x = -\frac{c}{a}</math>.
+Pada ruas kiri, kita dapat mengubahnya menjadi bentuk kuadrat dengan menambahkan <math display="inline">\left(\frac{b}{2a}\right)^2</math> pada kedua ruas.
+: <math>\left(x + \frac{b}{2a}\right)^2 = -\frac{c}{a} + \frac{b^2}{4a^2} </math>.
+Pada ruas kanan, penyebutnya disamakan. Kedua ruas diakarkuadratkan dan dikurangi dengan <math display="inline">-\frac{b}{a}</math> sehingga kita memperoleh rumus kuadrat.
+Melalui teknik yang serupa, terdapat cara sederhana dalam menurunkan rumus ini.<ref name="Hoehn1975">{{cite journal|last=Hoehn|first=Larry|year=1975|title=A More Elegant Method of Deriving the Quadratic Formula|journal=The Mathematics Teacher|volume=68|issue=5|page=442&ndash;443|doi=10.5951/MT.68.5.0442}}</ref> This derivation of the quadratic formula is ancient and was known in India at least as far back as 1025.<ref name="Smith1958">{{cite book|last=Smith|first=David E.|year=1958|title=History of Mathematics, Vol. II|publisher=Dover Publications|isbn=0486204308|page=446}}</ref> Compared with the derivation in standard usage, this alternate derivation avoids fractions and squared fractions until the last step and hence does not require a rearrangement after step 3 to obtain a common denominator in the right side.<ref name="Hoehn1975" />
-This derivation of the quadratic formula is ancient and was known in India at least as far back as 1025.<ref name="Smith1958">{{cite book|last=Smith|first=David E.|year=1958|title=History of Mathematics, Vol. II|publisher=Dover Publications|isbn=0486204308|page=446}}</ref> Compared with the derivation in standard usage, this alternate derivation avoids fractions and squared fractions until the last step and hence does not require a rearrangement after step 3 to obtain a common denominator in the right side.<ref name="Hoehn1975" />
 ==== Substitusi ====