Deret pangkat: Perbedaan antara revisi
JohnThorne (bicara | kontrib) Tidak ada ringkasan suntingan |
JohnThorne (bicara | kontrib) Tambahan informasi |
||
| Baris 1: | Baris 1: | ||
{{Kalkulus |deret}} |
|||
'''Deret pangkat''' (satu variabel) dalam [[matematika]] adalah [[deret tak terhingga]] dalam bentuk |
'''Deret pangkat''' (satu variabel) dalam [[matematika]] adalah [[deret tak terhingga]] dalam bentuk |
||
| Baris 12: | Baris 14: | ||
Deret pangkat biasanya ditemukan dalam [[analisis matematika]], tapi juga dapat ditemukan pada [[kombinatorika]] (dengan nama [[fungsi pembangkit]]), dan pada [[teknik elektro]] (dengan nama [[transformasi Z]]). |
Deret pangkat biasanya ditemukan dalam [[analisis matematika]], tapi juga dapat ditemukan pada [[kombinatorika]] (dengan nama [[fungsi pembangkit]]), dan pada [[teknik elektro]] (dengan nama [[transformasi Z]]). |
||
[[Image:Exp series.gif|right|thumb|[[:en:exponential function|Fungsi eksponensial]] (biru), dan jumlah ''n''+1 elemen pertama dari [[:en:Maclaurin series|deret pangkat Maclaurin]] (merah).]] |
|||
<!-- |
|||
==Examples==<!-- This section is linked from [[Complex plane]] --> |
|||
Any [[polynomial]] can be easily expressed as a power series around any center ''c'', although most of the coefficients will be zero since a power series has infinitely many terms by definition. For instance, the polynomial <math>f(x) = x^2 + 2x + 3</math> can be written as a power series around the center <math>c=0</math> as |
|||
::<math>f(x) = 3 + 2 x + 1 x^2 + 0 x^3 + 0 x^4 + \cdots \,</math> |
|||
or around the center <math>c=1</math> as |
|||
::<math>f(x) = 6 + 4 (x-1) + 1(x-1)^2</math><math> + 0(x-1)^3 + 0(x-1)^4 + \cdots \,</math> |
|||
or indeed around any other center ''c''.<ref>{{cite book|author=[[Howard Levi]]|title=Polynomials, Power Series, and Calculus|url=http://books.google.com/books?id=AcI-AAAAIAAJ|year=1967|publisher=Van Nostrand|pages=24}}</ref> One can view power series as being like "polynomials of infinite degree," although power series are not polynomials. |
|||
The [[geometric series]] formula |
|||
::<math> \frac{1}{1-x} = \sum_{n=0}^\infty x^n = 1 + x + x^2 + x^3 + \cdots,</math> |
|||
which is valid for <math>|x|<1</math>, is one of the most important examples of a power series, as are the exponential function |
|||
formula |
|||
::<math> e^x = \sum_{n=0}^\infty \frac{x^n}{n!} = 1 + x + \frac{x^2}{2!} + \frac{x^3}{3!} + \cdots,</math> |
|||
and the sine formula |
|||
::<math> \sin(x) = \sum_{n=0}^\infty \frac{(-1)^n x^{2n+1}}{(2n+1)!} = x - \frac{x^3}{3!} + \frac{x^5}{5!} - \frac{x^7}{7!}+\cdots,</math> |
|||
valid for all real x. |
|||
These power series are also examples of [[Taylor series]]. |
|||
Negative powers are not permitted in a power series, for instance <math>1 + x^{-1} + x^{-2} + \cdots</math> |
|||
is not considered a power series (although it is a [[Laurent series]]). Similarly, fractional powers such as <math>x^{1/2}</math> are not permitted (but see [[Puiseux series]]). The coefficients <math>a_n</math> are not allowed to depend on <math>x</math>, thus for instance: |
|||
:<math>\sin(x) x + \sin(2x) x^2 + \sin(3x) x^3 + \cdots \,</math> is not a power series. |
|||
==Radius of convergence== |
|||
A power series will converge for some values of the variable ''x'' and may diverge for others. All power series ''f''(''x'') in powers of (''x''-''c'') will converge at ''x'' = ''c''. (The correct value ''f''(''c'') = ''a''<sub>0</sub> requires interpreting the expression 0<sup>0</sup> as equal to 1.) If ''c'' is not the only convergent point, then there is always a number ''r'' with 0 < ''r'' ≤ ∞ such that the series converges whenever |''x'' − ''c''| < ''r'' and diverges whenever |''x'' − ''c''| > ''r''. The number ''r'' is called the '''[[radius of convergence]]''' of the power series; in general it is given as |
|||
:<math>r=\liminf_{n\to\infty} \left|a_n\right|^{-\frac{1}{n}}</math> |
|||
or, equivalently, |
|||
<math>r^{-1}=\limsup_{n\to\infty} \left|a_n\right|^{\frac{1}{n}}</math> |
|||
(this is the [[Cauchy–Hadamard theorem]]; see [[limit superior and limit inferior]] for an explanation of the notation). A fast way to compute it is |
|||
:<math>r^{-1}=\lim_{n\to\infty}\left|{a_{n+1}\over a_n}\right|</math> |
|||
if this limit exists. |
|||
The series [[absolute convergence|converges absolutely]] for |''x'' − ''c''| < ''r'' and [[uniform convergence|converges uniformly]] on every [[Compact space|compact]] [[subset]] of {''x'' : |''x'' − ''c''| < ''r''}. That is, the series is absolutely and [[compactly convergent]] on the interior of the disc of convergence. |
|||
For |''x'' − ''c''| = ''r'', we cannot make any general statement on whether the series converges or diverges. However, for the case of real variables, [[Abel's theorem]] states that the sum of the series is continuous at ''x'' if the series converges at ''x''. In the case of complex variables, we can only claim continuity along the line segment starting at ''c'' and ending at ''x''. |
|||
--> |
|||
== Operasi pada deret pangkat == |
|||
=== Penjumlahan dan pengurangan === |
|||
Bilamana dua fungsi ''f'' dan ''g'' didekomposisi menjadi deret pangkat sekitar pusat ''c'' yang sama, deret pangkat dari jumlah atau selisih kedua fungsi itu dapat dihitung masing-masing dengan penjumlahan atau pengurangan. Yaitu, jika: |
|||
:<math>f(x) = \sum_{n=0}^\infty a_n (x-c)^n</math> |
|||
:<math>g(x) = \sum_{n=0}^\infty b_n (x-c)^n</math> |
|||
maka |
|||
:<math>f(x)\pm g(x) = \sum_{n=0}^\infty (a_n \pm b_n) (x-c)^n.</math> |
|||
=== Perkalian dan pembagian === |
|||
Dengan definisi yang sama seperti di atas, hasil kali dan hasil bagi deret pangkat dari kedua fungsi itu dapat diperoleh sebagai berikut: |
|||
:<math> f(x)g(x) = \left(\sum_{n=0}^\infty a_n (x-c)^n\right)\left(\sum_{n=0}^\infty b_n (x-c)^n\right)</math> |
|||
:<math> = \sum_{i=0}^\infty \sum_{j=0}^\infty a_i b_j (x-c)^{i+j}</math> |
|||
:<math> = \sum_{n=0}^\infty \left(\sum_{i=0}^n a_i b_{n-i}\right) (x-c)^n.</math> |
|||
Urutan <math>m_n = \sum_{i=0}^n a_i b_{n-i}</math> dikenal sebagai [[:en:convolution|konvolusi]] urutan <math>a_n</math> dan <math>b_n</math>. |
|||
Untuk pembagian, perhatikan: |
|||
:<math> {f(x)\over g(x)} = {\sum_{n=0}^\infty a_n (x-c)^n\over\sum_{n=0}^\infty b_n (x-c)^n} = \sum_{n=0}^\infty d_n (x-c)^n</math> |
|||
:<math> f(x) = \left(\sum_{n=0}^\infty b_n (x-c)^n\right)\left(\sum_{n=0}^\infty d_n (x-c)^n\right)</math> |
|||
dan kemudian gunakan koefisien-koefisien pembanding di atas. |
|||
=== Diferensiasi dan integrasi === |
|||
Bilamana suatu fungsi dinyatakan sebagai deret pangkat, maka fungsi itu [[derivatif|dapat dihitung diferensialnya]] pada [[:en:interior (topology)|interior]] ranah konvergensi. Dapat dihitung [[derivatif|diferensial]] dan [[integral]] dengan mudah dengan mengerjakan setiap elemen secara terpisah: |
|||
::<math> |
|||
f^\prime (x) = \sum_{n=1}^\infty a_n n \left( x-c \right)^{n-1}= \sum_{n=0}^\infty a_{n+1} \left(n+1 \right) \left( x-c \right)^{n} |
|||
</math> |
|||
::<math> |
|||
\int f(x)\,dx = \sum_{n=0}^\infty \frac{a_n \left( x-c \right)^{n+1}} {n+1} + k = \sum_{n=1}^\infty \frac{a_{n-1} \left( x-c \right)^{n}} {n} + k. |
|||
</math> |
|||
Kedua deret ini memiliki jari-jari konvergensi yang sama dengan deret asalnya. |
|||
<!-- |
|||
== Fungsi analitik == |
|||
A function ''f'' defined on some [[open set|open subset]] ''U'' of '''R''' or '''C''' is called '''analytic''' if it is locally given by a convergent power series. This means that every ''a'' ∈ ''U'' has an open [[neighborhood (topology)|neighborhood]] ''V'' ⊆ ''U'', such that there exists a power series with center ''a'' which converges to ''f''(''x'') for every ''x'' ∈ ''V''. |
|||
Every power series with a positive radius of convergence is analytic on the [[topological interior|interior]] of its region of convergence. All [[holomorphic function]]s are complex-analytic. Sums and products of analytic functions are analytic, as are quotients as long as the denominator is non-zero. |
|||
If a function is analytic, then it is infinitely often differentiable, but in the real case the converse is not generally true. For an analytic function, the coefficients ''a''<sub>''n''</sub> can be computed as |
|||
::<math> |
|||
a_n = \frac {f^{\left( n \right)}\left( c \right)} {n!} |
|||
</math> |
|||
where <math>f^{(n)}(c)</math> denotes the ''n''th derivative of ''f'' at ''c'', and <math>f^{(0)}(c) = f(c)</math>. This means that every analytic function is locally represented by its [[Taylor series]]. |
|||
The global form of an analytic function is completely determined by its local behavior in the following sense: if ''f'' and ''g'' are two analytic functions defined on the same [[connectedness|connected]] open set ''U'', and if there exists an element ''c''∈''U'' such that ''f''<sup> (''n'')</sup>(''c'') = ''g''<sup> (''n'')</sup>(''c'') for all ''n'' ≥ 0, then ''f''(''x'') = ''g''(''x'') for all ''x'' ∈ ''U''. |
|||
If a power series with radius of convergence ''r'' is given, one can consider [[analytic continuation]]s of the series, i.e. analytic functions ''f'' which are defined on larger sets than { ''x'' : |''x'' − ''c''| < ''r'' } and agree with the given power series on this set. The number ''r'' is maximal in the following sense: there always exists a [[complex number]] ''x'' with |''x'' − ''c''| = ''r'' such that no analytic continuation of the series can be defined at ''x''. |
|||
The power series expansion of the [[inverse function]] of an analytic function can be determined using the [[Lagrange inversion theorem]]. |
|||
== Formal power series == |
|||
{{main|Formal power series}} |
|||
In [[abstract algebra]], one attempts to capture the essence of power series without being restricted to the [[field (mathematics)|field]]s of real and complex numbers, and without the need to talk about convergence. This leads to the concept of [[formal power series]], a concept of great utility in [[algebraic combinatorics]]. |
|||
== Power series in several variables == |
|||
An extension of the theory is necessary for the purposes of [[multivariable calculus]]. A '''power series''' is here defined to be an infinite series of the form |
|||
::<math> |
|||
f(x_1,\dots,x_n) = \sum_{j_1,\dots,j_n = 0}^{\infty}a_{j_1,\dots,j_n} \prod_{k=1}^n \left(x_k - c_k \right)^{j_k}, |
|||
</math> |
|||
where ''j'' = (''j''<sub>1</sub>, ..., ''j''<sub>''n''</sub>) is a vector of natural numbers, the coefficients |
|||
''a''<sub>(''j<sub>1</sub>,...,j<sub>n</sub>'')</sub> are usually real or complex numbers, and the center ''c'' = (''c''<sub>1</sub>, ..., ''c''<sub>''n''</sub>) and argument ''x'' = (''x''<sub>1</sub>, ..., ''x''<sub>''n''</sub>) are usually real or complex vectors. In the more convenient [[multi-index]] notation this can be written |
|||
::<math> |
|||
f(x) = \sum_{\alpha \in \mathbb{N}^n} a_{\alpha} \left(x - c \right)^{\alpha}. |
|||
</math> |
|||
The theory of such series is trickier than for single-variable series, with more complicated regions of convergence. For instance, the power series <math> \sum_{n=0}^\infty x_1^n x_2^n</math> is absolutely convergent in the set <math>\{ (x_1,x_2): |x_1 x_2| < 1\}</math> between two hyperbolas. (This is an example of a ''log-convex set'', in the sense that the set of points <math>(\log |x_1|, \log |x_2|)</math>, where <math>(x_1,x_2)</math> lies in the above region, is a convex set. More generally, one can show that when c=0, the interior of the region of absolute convergence is always a log-convex set in this sense.) On the other hand, in the interior of this region of convergence one may differentiate and integrate under the series sign, just as one may with ordinary power series. |
|||
--> |
|||
== Tingkatan deret pangkat == |
|||
Misalkan α adalah suatu multi-indeks untuk deret pangkat''f''(''x''<sub>1</sub>, ''x''<sub>2</sub>, …, ''x''<sub>''n''</sub>). '''Tingkata''' (''order'') dari deret pangkat ''f'' didefinisikan sebagai nilai terkecil |α| sedemikian sehingga ''a''<sub>α</sub> ≠ 0, atau 0 jika ''f'' ≡ 0. Khususnya, untuk deret pangkat ''f''(''x'') dalam variabel tunggal ''x'', tingkatan ''f'' adalah pangkat terkecil dari ''x'' dengan koefisien bukan-nol. Definisi ini mudah dikembangkan ke [[:en:Laurent series|deret Laurent]]. |
|||
== Lihat pula == |
== Lihat pula == |
||
* [[Deret (matematika)]] |
* [[Deret (matematika)]] |
||
<!--* [[Flat function]] |
|||
* [[Linear approximation]] |
|||
* [[Random variable]]--> |
|||
==Referensi== |
|||
{{Reflist}} |
|||
== Pranala luar == |
== Pranala luar == |
||
*{{SpringerEOM|title=Power series|id=Power_series&oldid=15309|last=Solomentsev|first=E.D.}} |
|||
* {{MathWorld | urlname= FormalPowerSeries | title= Formal Power Series }} |
* {{MathWorld | urlname= FormalPowerSeries | title= Formal Power Series }} |
||
* {{MathWorld | urlname= PowerSeries | title= Power Series }} |
* {{MathWorld | urlname= PowerSeries | title= Power Series }} |
||
Revisi per 15 Januari 2015 06.11
| Kalkulus |
|---|
Deret pangkat (satu variabel) dalam matematika adalah deret tak terhingga dalam bentuk
dengan an melambangkan koefisien suku ke-n, c adalah konstanta dan x berubah-ubah di sekitar c (karena alasan ini kadang-kadang deret seperti ini dikatakan berpusat di c). Deret ini biasanya berupa deret Taylor dari suatu fungsi.
Pada banyak keadaan c sama dengan nol, contohnya pada deret Maclaurin. Dalam hal tersebut deret pangkat mengambil bentuk yang lebih sederhana:
Deret pangkat biasanya ditemukan dalam analisis matematika, tapi juga dapat ditemukan pada kombinatorika (dengan nama fungsi pembangkit), dan pada teknik elektro (dengan nama transformasi Z).
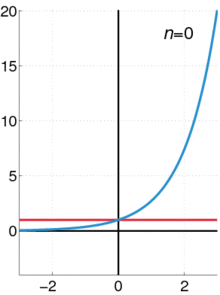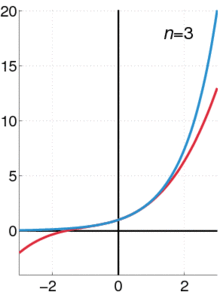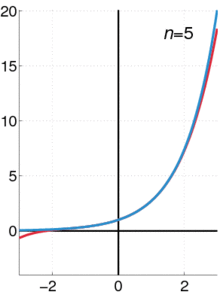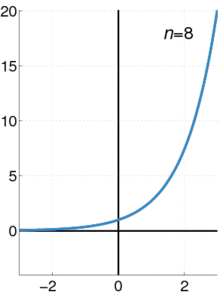
Any polynomial can be easily expressed as a power series around any center c, although most of the coefficients will be zero since a power series has infinitely many terms by definition. For instance, the polynomial can be written as a power series around the center as
or around the center as
or indeed around any other center c.[1] One can view power series as being like "polynomials of infinite degree," although power series are not polynomials.
The geometric series formula
which is valid for , is one of the most important examples of a power series, as are the exponential function formula
and the sine formula
valid for all real x. These power series are also examples of Taylor series.
Negative powers are not permitted in a power series, for instance is not considered a power series (although it is a Laurent series). Similarly, fractional powers such as are not permitted (but see Puiseux series). The coefficients are not allowed to depend on , thus for instance:
- is not a power series.
Radius of convergence
A power series will converge for some values of the variable x and may diverge for others. All power series f(x) in powers of (x-c) will converge at x = c. (The correct value f(c) = a0 requires interpreting the expression 00 as equal to 1.) If c is not the only convergent point, then there is always a number r with 0 < r ≤ ∞ such that the series converges whenever |x − c| < r and diverges whenever |x − c| > r. The number r is called the radius of convergence of the power series; in general it is given as
or, equivalently,
(this is the Cauchy–Hadamard theorem; see limit superior and limit inferior for an explanation of the notation). A fast way to compute it is
if this limit exists.
The series converges absolutely for |x − c| < r and converges uniformly on every compact subset of {x : |x − c| < r}. That is, the series is absolutely and compactly convergent on the interior of the disc of convergence.
For |x − c| = r, we cannot make any general statement on whether the series converges or diverges. However, for the case of real variables, Abel's theorem states that the sum of the series is continuous at x if the series converges at x. In the case of complex variables, we can only claim continuity along the line segment starting at c and ending at x. -->
Operasi pada deret pangkat
Penjumlahan dan pengurangan
Bilamana dua fungsi f dan g didekomposisi menjadi deret pangkat sekitar pusat c yang sama, deret pangkat dari jumlah atau selisih kedua fungsi itu dapat dihitung masing-masing dengan penjumlahan atau pengurangan. Yaitu, jika:
maka
Perkalian dan pembagian
Dengan definisi yang sama seperti di atas, hasil kali dan hasil bagi deret pangkat dari kedua fungsi itu dapat diperoleh sebagai berikut:
Urutan dikenal sebagai konvolusi urutan dan .
Untuk pembagian, perhatikan:
dan kemudian gunakan koefisien-koefisien pembanding di atas.
Diferensiasi dan integrasi
Bilamana suatu fungsi dinyatakan sebagai deret pangkat, maka fungsi itu dapat dihitung diferensialnya pada interior ranah konvergensi. Dapat dihitung diferensial dan integral dengan mudah dengan mengerjakan setiap elemen secara terpisah:
Kedua deret ini memiliki jari-jari konvergensi yang sama dengan deret asalnya.
Tingkatan deret pangkat
Misalkan α adalah suatu multi-indeks untuk deret pangkatf(x1, x2, …, xn). Tingkata (order) dari deret pangkat f didefinisikan sebagai nilai terkecil |α| sedemikian sehingga aα ≠ 0, atau 0 jika f ≡ 0. Khususnya, untuk deret pangkat f(x) dalam variabel tunggal x, tingkatan f adalah pangkat terkecil dari x dengan koefisien bukan-nol. Definisi ini mudah dikembangkan ke deret Laurent.
Lihat pula
Referensi
- ^ Howard Levi (1967). Polynomials, Power Series, and Calculus. Van Nostrand. hlm. 24.
Pranala luar
- Solomentsev, E.D. (2001) [1994], "Power series", dalam Hazewinkel, Michiel, Encyclopedia of Mathematics, Springer Science+Business Media B.V. / Kluwer Academic Publishers, ISBN 978-1-55608-010-4
- (Inggris) Weisstein, Eric W. "Formal Power Series". MathWorld.
- (Inggris) Weisstein, Eric W. "Power Series". MathWorld.
- (Inggris)Modul deret pangkat kompleks oleh John H. Mathews
- (Inggris)Pangkat bilangan kompleks oleh Michael Schreiber, Wolfram Demonstrations Project.
































