Pengguna:Kekavigi/bak pasir 2: Perbedaan antara revisi
k hanya noindex Tag: Penggantian Pengembalian manual Suntingan visualeditor-wikitext |
draf: mencoba menggabungkan en:Square number ke en:Square_(algebra) |
||
| Baris 1: | Baris 1: | ||
{{NOINDEX}}{{short description|Product of an integer with itself}} |
|||
{{NOINDEX}} |
|||
[[Berkas:Square_number_16_as_sum_of_gnomons.svg|jmpl|Bilangan kuadrat 16 dapat dilihat sebagai penjumlahan empat bilangan ganjil (positif) pertama: 1+3+5+7.]] |
|||
Dalam matematika, '''bilangan kuadrat''' atau '''kuadrat sempurna''' adalah bilangan bulat yang merupakan kuadrat dari suatu bilangan bulat lainnya. Dalam kata lain, bilangan yang dihasilkan dari perkalian suatu bilangan bulat dengan dirinya sendiri. Sebagai contoh, 9 adalah bilangan kuadrat karena bernilai sama dengan <math>3^2</math>, dan dapat ditulis sebagai <math>3\times3</math>. Dalam sistem bilangan real, definisi lain untuk bilangan kuadrat adalah bilangan yang akar kuadratnya merupakan bilangan bulat. Sebagai contoh, 9 adalah bilangan kuadrat karena <math>\sqrt{9} = 3</math> merupakan bilangan bulat. |
|||
Notasi yang umum untuk kuadrat suatu bilangan <math>n</math> bukan bentuk perkalian <math>n\times n</math>, melainkan bentuk perpangkatan <math>n^2</math>, umum dilafalkan sebagai "<math>n</math> kuadrat". |
|||
Konsep bilangan kuadrat dapat diperumum ke beberapa sistem bilangan lainnya. Pada sistem bilangan rasional, bilangan kuadrat adalah bilangan yang dapat dituliskan sebagai rasio dari dua bilangan bulat kuadrat; sebagai contoh, <math>\textstyle \frac{4}{9} = \left(\frac{2}{3}\right)^2</math>. |
|||
Bilangan bulat positif yang tidak memiliki pembagi berupa bilangan kuadrat selain 1, disebut sebagai bilangan bebas kuadrat. |
|||
== Contoh == |
|||
Ada <math>\lfloor \sqrt{m} \rfloor</math> bilangan kuadrat diantara 1 sampai (dan termasuk) {{mvar|m}}, dengan notasi <math>\lfloor x \rfloor</math> menyatakan fungsi lantai dari <math>x</math>. |
|||
Selisih antara sebarang bilangan kuadrat dengan pendahulunya dapat dituliskan lewat hubungan <math>n^2 - (n-1)^2 = 2n-1</math>. |
|||
== Sifat == |
|||
Bilangan <math>m</math> adalah bilangan kuadrat jika dan hanya jika <math>m</math> titik dapat disusun sebagai sebuah persegi seperti berikut: |
|||
{| cellpadding="8" |
|||
|{{bigmath|1=''m'' = 1<sup>2</sup> = 1}} |
|||
|[[Berkas:Square_number_1.png]] |
|||
|- |
|||
|{{bigmath|1=''m'' = 2<sup>2</sup> = 4}} |
|||
|[[Berkas:Square_number_4.png]] |
|||
|- |
|||
|{{bigmath|1=''m'' = 3<sup>2</sup> = 9}} |
|||
|[[Berkas:Square_number_9.png]] |
|||
|- |
|||
|{{bigmath|1=''m'' = 4<sup>2</sup> = 16}} |
|||
|[[Berkas:Square_number_16.png]] |
|||
|- |
|||
|{{bigmath|1=''m'' = 5<sup>2</sup> = 25}} |
|||
|[[Berkas:Square_number_25.png]] |
|||
|} |
|||
Bentuk untuk bilangan kuadrat ke-<math>n</math> adalah <math>n^2</math>. Bentuk ini sama dengan jumlah <math>n</math> bilangan ganjil (positif) pertama. Seperti pada gambar di atas, bilangan kuadrat baru dapat dihasilkan dari bilangan kuadrat dengan menambahkan sejumlah ganjil titik baru (ditandai dengan warna ungu). Secara matematis, visualisasi tersebut menunjukkan hubungan <math display="block">n^2 = \sum_{k=1}^n (2k-1).</math> Sebagai contoh, {{math|1=5<sup>2</sup> = 25 = 1 + 3 + 5 + 7 + 9}}. |
|||
[[Berkas:The_sum_of_the_first_n_odd_integers_is_n²._1+3+5+...+(2n-1)=n²..gif|jmpl|The sum of the first ''n'' odd integers is ''n''<sup>2</sup>. {{nowrap|1 + 3 + 5 + ... + (2''n'' − 1) {{=}} ''n''<sup>2</sup>}}. Animated 3D visualization on a tetrahedron.]] |
|||
There are several [[Recursion#Recursion in mathematics|recursive]] methods for computing square numbers. For example, the {{mvar|n}}th square number can be computed from the previous square by {{math|''n''{{sup|2}} {{=}} (''n'' − 1){{sup|2}} + (''n'' − 1) + n {{=}} (''n'' − 1){{sup|2}} + (2''n'' − 1)}}. Alternatively, the {{mvar|n}}th square number can be calculated from the previous two by doubling the {{math|(''n'' − 1)}}th square, subtracting the {{math|(''n'' − 2)}}th square number, and adding 2, because {{math|1=''n''<sup>2</sup> = 2(''n'' − 1)<sup>2</sup> − (''n'' − 2)<sup>2</sup> + 2}}. For example, |
|||
: {{math|1=2 × 5<sup>2</sup> − 4<sup>2</sup> + 2 = 2 × 25 − 16 + 2 = 50 − 16 + 2 = 36 = 6<sup>2</sup>}}. |
|||
The square minus one of a number {{mvar|m}} is always the product of <math>m - 1</math> and <math>m + 1;</math> that is,<math display="block">m^2-1=(m-1)(m+1).</math>For example, since {{Math|1=7<sup>2</sup> = 49}}, one has <math>6 \times 8 = 48</math>. Since a [[prime number]] has factors of only {{Math|1}} and itself, and since {{Math|1=''m'' = 2}} is the only non-zero value of {{Math|''m''}} to give a factor of {{Math|1}} on the right side of the equation above, it follows that {{math|3}} is the only prime number one less than a square ({{math|1=3 = 2{{sup|2}} − 1}}). |
|||
More generally, the difference of the squares of two numbers is the product of their sum and their difference. That is,<math display="block">a^2-b^2=(a+b)(a-b)</math>This is the [[Difference of two squares|difference-of-squares formula]], which can be useful for mental arithmetic: for example, {{math|47 × 53}} can be easily computed as {{math|1=50<sup>2</sup> − 3<sup>2</sup> = 2500 − 9 = 2491}}. |
|||
A square number is also the sum of two consecutive [[Triangular number|triangular numbers]]. The sum of two consecutive square numbers is a [[centered square number]]. Every odd square is also a [[centered octagonal number]]. |
|||
Another property of a square number is that (except 0) it has an odd number of positive divisors, while other natural numbers have an [[Parity (mathematics)|even number]] of positive divisors. An integer root is the only divisor that pairs up with itself to yield the square number, while other divisors come in pairs. |
|||
[[Lagrange's four-square theorem]] states that any positive integer can be written as the sum of four or fewer perfect squares. Three squares are not sufficient for numbers of the form {{math|4<sup>''k''</sup>(8''m'' + 7)}}. A positive integer can be represented as a sum of two squares precisely if its [[prime factorization]] contains no odd powers of primes of the form {{math|4''k'' + 3}}. This is generalized by [[Waring's problem]]. |
|||
In [[base 10]], a square number can end only with digits 0, 1, 4, 5, 6 or 9, as follows: |
|||
* if the last digit of a number is 0, its square ends in 00; |
|||
* if the last digit of a number is 1 or 9, its square ends in an even digit followed by a 1; |
|||
* if the last digit of a number is 2 or 8, its square ends in an even digit followed by a 4; |
|||
* if the last digit of a number is 3 or 7, its square ends in an even digit followed by a 9; |
|||
* if the last digit of a number is 4 or 6, its square ends in an odd digit followed by a 6; and |
|||
* if the last digit of a number is 5, its square ends in 25. |
|||
In [[base 12]], a square number can end only with square digits (like in base 12, a [[prime number]] can end only with prime digits or 1), that is, 0, 1, 4 or 9, as follows: |
|||
* if a number is divisible both by 2 and by 3 (that is, divisible by 6), its square ends in 0, and its preceding digit must be 0 or 3; |
|||
* if a number is divisible neither by 2 nor by 3, its square ends in 1, and its preceding digit must be even; |
|||
* if a number is divisible by 2, but not by 3, its square ends in 4, and its preceding digit must be 0, 1, 4, 5, 8, or 9; and |
|||
* if a number is not divisible by 2, but by 3, its square ends in 9, and its preceding digit must be 0 or 6. |
|||
Similar rules can be given for other bases, or for earlier digits (the tens instead of the units digit, for example).{{citation needed|date=March 2016}} All such rules can be proved by checking a fixed number of cases and using [[modular arithmetic]]. |
|||
In general, if a [[Prime number|prime]] {{mvar|p}} divides a square number {{mvar|m}} then the square of {{mvar|p}} must also divide {{mvar|m}}; if {{mvar|p}} fails to divide {{math|{{sfrac|''m''|''p''}}}}, then {{mvar|m}} is definitely not square. Repeating the divisions of the previous sentence, one concludes that every prime must divide a given perfect square an even number of times (including possibly 0 times). Thus, the number {{mvar|m}} is a square number if and only if, in its [[Canonical representation of a positive integer|canonical representation]], all exponents are even. |
|||
Squarity testing can be used as alternative way in [[factorization]] of large numbers. Instead of testing for divisibility, test for squarity: for given {{mvar|m}} and some number {{mvar|k}}, if {{math|''k''<sup>2</sup> − ''m''}} is the square of an integer {{mvar|n}} then {{math|''k'' − ''n''}} divides {{mvar|m}}. (This is an application of the factorization of a [[difference of two squares]].) For example, {{math|100<sup>2</sup> − 9991}} is the square of 3, so consequently {{math|100 − 3}} divides 9991. This test is deterministic for odd divisors in the range from {{math|''k'' − ''n''}} to {{math|''k'' + ''n''}} where {{mvar|k}} covers some range of natural numbers <math>k \geq \sqrt{m}.</math> |
|||
A square number cannot be a [[perfect number]]. |
|||
The sum of the ''n'' first square numbers is<math display="block">\sum_{n=0}^N n^2 = 0^2 + 1^2 + 2^2 + 3^2 + 4^2 + \cdots + N^2 = \frac{N(N+1)(2N+1)}{6}.</math>The first values of these sums, the [[Square pyramidal number|square pyramidal numbers]], are: {{OEIS|id=A000330}}<blockquote>0, 1, 5, 14, 30, 55, 91, 140, 204, 285, 385, 506, 650, 819, 1015, 1240, 1496, 1785, 2109, 2470, 2870, 3311, 3795, 4324, 4900, 5525, 6201...</blockquote> |
|||
[[Berkas:Proofwithoutwords.svg|jmpl|100x100px|Proof without words for the sum of odd numbers theorem]] |
|||
The sum of the first odd integers, beginning with one, is a perfect square: 1, 1 + 3, 1 + 3 + 5, 1 + 3 + 5 + 7, etc. This explains [[Galileo's law of odd numbers]]: if a body falling from rest covers one unit of distance in the first arbitrary time interval, it covers 3, 5, 7, etc., units of distance in subsequent time intervals of the same length. From <math>s=ut+\tfrac{1}{2}at^2</math>, for {{Math|1=''u'' = 0}} and constant {{Math|''a''}} (acceleration due to gravity without air resistance); so {{Math|''s''}} is proportional to {{Math|''t''<sup>2</sup>}}, and the distance from the starting point are consecutive squares for integer values of time elapsed.<ref>{{Cite book|last1=Olenick|first1=Richard P.|last2=Apostol|first2=Tom M.|last3=Goodstein|first3=David L.|date=2008-01-14|url=https://books.google.com/books?id=xMWwTpn53KsC&pg=PA18|title=The Mechanical Universe: Introduction to Mechanics and Heat|publisher=Cambridge University Press|isbn=978-0-521-71592-8|pages=18|language=en}}</ref> |
|||
The sum of the ''n'' first [[Cube (algebra)|cubes]] is the square of the sum of the ''n'' first positive integers; this is [[Nicomachus's theorem]]. |
|||
All fourth powers, sixth powers, eighth powers and so on are perfect squares. |
|||
A unique relationship with triangular numbers <math>T_n</math> is:<math display="block">(T_n)^2 + (T_{n+1})^2 = T_{(n+1)^2}</math> |
|||
== Odd and even square numbers ==<!-- This section is linked from [[Irrational number]] --> |
|||
Squares of even numbers are even, and are divisible by 4, since {{Math|1=(2''n'')<sup>2</sup> = 4''n''<sup>2</sup>}}. Squares of odd numbers are odd, and are congruent to 1 [[Modular arithmetic|modulo]] 8, since {{Math|1=(2''n'' + 1)<sup>2</sup> = 4''n''(''n'' + 1) + 1}}, and {{Math|''n''(''n'' + 1)}} is always even. In other words, all odd square numbers have a remainder of 1 when divided by 8. |
|||
Every odd perfect square is a [[centered octagonal number]]. The difference between any two odd perfect squares is a multiple of 8. The difference between 1 and any higher odd perfect square always is eight times a triangular number, while the difference between 9 and any higher odd perfect square is eight times a triangular number minus eight. Since all triangular numbers have an odd factor, but no two values of {{math|2<sup>''n''</sup>}} differ by an amount containing an odd factor, the only perfect square of the form {{math|2<sup>''n''</sup> − 1}} is 1, and the only perfect square of the form {{math|2<sup>''n''</sup> + 1}} is 9. |
|||
== Special cases == |
|||
* If the number is of the form {{math|''m''5}} where {{math|''m''}} represents the preceding digits, its square is {{math|''n''25}} where {{math|1=''n'' = ''m''(''m'' + 1)}} and represents digits before 25. For example, the square of 65 can be calculated by {{math|1=''n'' = 6 × (6 + 1) = 42}} which makes the square equal to 4225. |
|||
* If the number is of the form {{math|''m''0}} where {{math|''m''}} represents the preceding digits, its square is {{math|''n''00}} where {{math|1=''n'' = ''m''<sup>2</sup>}}. For example, the square of 70 is 4900. |
|||
* If the number has two digits and is of the form {{math|5''m''}} where {{math|''m''}} represents the units digit, its square is {{math|''aabb''}} where {{math|1=''aa'' = 25 + ''m''}} and {{math|1=''bb'' = ''m''<sup>2</sup>}}. For example, to calculate the square of 57, {{Math|1=''m'' = 7}} and {{Math|1=25 + 7 = 32}} and {{Math|1=7<sup>2</sup> = 49}}, so {{Math|1=57<sup>2</sup> = 3249}}. |
|||
* If the number ends in 5, its square will end in 5; similarly for ending in 25, 625, 0625, 90625, ... 8212890625, etc. If the number ends in 6, its square will end in 6, similarly for ending in 76, 376, 9376, 09376, ... 1787109376. For example, the square of 55376 is 3066501376, both ending in ''376''. (The numbers 5, 6, 25, 76, etc. are called [[Automorphic number|automorphic numbers]]. They are sequence [[oeis:A003226|A003226]] in the [[On-Line Encyclopedia of Integer Sequences|OEIS]].<ref>{{Cite OEIS|A003226|Automorphic numbers: n^2 ends with n.}}</ref>) |
|||
* In base 10, the last two digits of square numbers follow a repeating pattern mirrored symmetrical around multiples of 25. In the example of 24 and 26, both 1 off from 25, {{Math|1=24<sup>2</sup> = 576}} and {{Math|1=26<sup>2</sup> = 676}}, both ending in 76. In general, <math display="inline">(25n+x)^2-(25n-x)^2=100nx</math>. An analogous pattern applies for the last 3 digits around multiples of 250, and so on. As a consequence, of the 100 possible last 2 digits, only 22 of them occur among square numbers (since 00 and 25 are repeated). |
|||
== Notes == |
|||
{{reflist}} |
|||
== Further reading == |
|||
* [[J.H. Conway|Conway, J. H.]] and [[R.K. Guy|Guy, R. K.]] ''The Book of Numbers''. New York: Springer-Verlag, pp. 30–32, 1996. {{isbn|0-387-97993-X}} |
|||
* Kiran Parulekar. ''Amazing Properties of Squares and Their Calculations''. Kiran Anil Parulekar, 2012 https://books.google.com/books?id=njEtt7rfexEC |
|||
Revisi per 15 September 2023 04.26

Dalam matematika, bilangan kuadrat atau kuadrat sempurna adalah bilangan bulat yang merupakan kuadrat dari suatu bilangan bulat lainnya. Dalam kata lain, bilangan yang dihasilkan dari perkalian suatu bilangan bulat dengan dirinya sendiri. Sebagai contoh, 9 adalah bilangan kuadrat karena bernilai sama dengan , dan dapat ditulis sebagai . Dalam sistem bilangan real, definisi lain untuk bilangan kuadrat adalah bilangan yang akar kuadratnya merupakan bilangan bulat. Sebagai contoh, 9 adalah bilangan kuadrat karena merupakan bilangan bulat.
Notasi yang umum untuk kuadrat suatu bilangan bukan bentuk perkalian , melainkan bentuk perpangkatan , umum dilafalkan sebagai " kuadrat".
Konsep bilangan kuadrat dapat diperumum ke beberapa sistem bilangan lainnya. Pada sistem bilangan rasional, bilangan kuadrat adalah bilangan yang dapat dituliskan sebagai rasio dari dua bilangan bulat kuadrat; sebagai contoh, .
Bilangan bulat positif yang tidak memiliki pembagi berupa bilangan kuadrat selain 1, disebut sebagai bilangan bebas kuadrat.
Contoh
Ada bilangan kuadrat diantara 1 sampai (dan termasuk) m, dengan notasi menyatakan fungsi lantai dari .
Selisih antara sebarang bilangan kuadrat dengan pendahulunya dapat dituliskan lewat hubungan .
Sifat
Bilangan adalah bilangan kuadrat jika dan hanya jika titik dapat disusun sebagai sebuah persegi seperti berikut:
| m = 12 = 1 | |
| m = 22 = 4 | |
| m = 32 = 9 | |
| m = 42 = 16 | 
|
| m = 52 = 25 | 
|
Bentuk untuk bilangan kuadrat ke- adalah . Bentuk ini sama dengan jumlah bilangan ganjil (positif) pertama. Seperti pada gambar di atas, bilangan kuadrat baru dapat dihasilkan dari bilangan kuadrat dengan menambahkan sejumlah ganjil titik baru (ditandai dengan warna ungu). Secara matematis, visualisasi tersebut menunjukkan hubungan
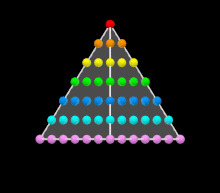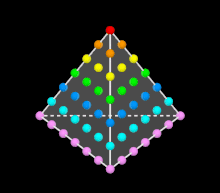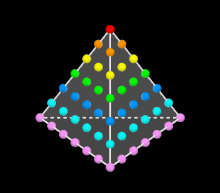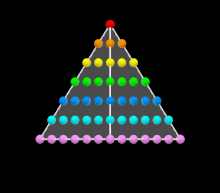
There are several recursive methods for computing square numbers. For example, the nth square number can be computed from the previous square by n2 = (n − 1)2 + (n − 1) + n = (n − 1)2 + (2n − 1). Alternatively, the nth square number can be calculated from the previous two by doubling the (n − 1)th square, subtracting the (n − 2)th square number, and adding 2, because n2 = 2(n − 1)2 − (n − 2)2 + 2. For example,
- 2 × 52 − 42 + 2 = 2 × 25 − 16 + 2 = 50 − 16 + 2 = 36 = 62.
The square minus one of a number m is always the product of and that is,
More generally, the difference of the squares of two numbers is the product of their sum and their difference. That is,
A square number is also the sum of two consecutive triangular numbers. The sum of two consecutive square numbers is a centered square number. Every odd square is also a centered octagonal number.
Another property of a square number is that (except 0) it has an odd number of positive divisors, while other natural numbers have an even number of positive divisors. An integer root is the only divisor that pairs up with itself to yield the square number, while other divisors come in pairs.
Lagrange's four-square theorem states that any positive integer can be written as the sum of four or fewer perfect squares. Three squares are not sufficient for numbers of the form 4k(8m + 7). A positive integer can be represented as a sum of two squares precisely if its prime factorization contains no odd powers of primes of the form 4k + 3. This is generalized by Waring's problem.
In base 10, a square number can end only with digits 0, 1, 4, 5, 6 or 9, as follows:
- if the last digit of a number is 0, its square ends in 00;
- if the last digit of a number is 1 or 9, its square ends in an even digit followed by a 1;
- if the last digit of a number is 2 or 8, its square ends in an even digit followed by a 4;
- if the last digit of a number is 3 or 7, its square ends in an even digit followed by a 9;
- if the last digit of a number is 4 or 6, its square ends in an odd digit followed by a 6; and
- if the last digit of a number is 5, its square ends in 25.
In base 12, a square number can end only with square digits (like in base 12, a prime number can end only with prime digits or 1), that is, 0, 1, 4 or 9, as follows:
- if a number is divisible both by 2 and by 3 (that is, divisible by 6), its square ends in 0, and its preceding digit must be 0 or 3;
- if a number is divisible neither by 2 nor by 3, its square ends in 1, and its preceding digit must be even;
- if a number is divisible by 2, but not by 3, its square ends in 4, and its preceding digit must be 0, 1, 4, 5, 8, or 9; and
- if a number is not divisible by 2, but by 3, its square ends in 9, and its preceding digit must be 0 or 6.
Similar rules can be given for other bases, or for earlier digits (the tens instead of the units digit, for example).[butuh rujukan] All such rules can be proved by checking a fixed number of cases and using modular arithmetic.
In general, if a prime p divides a square number m then the square of p must also divide m; if p fails to divide mp, then m is definitely not square. Repeating the divisions of the previous sentence, one concludes that every prime must divide a given perfect square an even number of times (including possibly 0 times). Thus, the number m is a square number if and only if, in its canonical representation, all exponents are even.
Squarity testing can be used as alternative way in factorization of large numbers. Instead of testing for divisibility, test for squarity: for given m and some number k, if k2 − m is the square of an integer n then k − n divides m. (This is an application of the factorization of a difference of two squares.) For example, 1002 − 9991 is the square of 3, so consequently 100 − 3 divides 9991. This test is deterministic for odd divisors in the range from k − n to k + n where k covers some range of natural numbers
A square number cannot be a perfect number.
The sum of the n first square numbers is
0, 1, 5, 14, 30, 55, 91, 140, 204, 285, 385, 506, 650, 819, 1015, 1240, 1496, 1785, 2109, 2470, 2870, 3311, 3795, 4324, 4900, 5525, 6201...

The sum of the first odd integers, beginning with one, is a perfect square: 1, 1 + 3, 1 + 3 + 5, 1 + 3 + 5 + 7, etc. This explains Galileo's law of odd numbers: if a body falling from rest covers one unit of distance in the first arbitrary time interval, it covers 3, 5, 7, etc., units of distance in subsequent time intervals of the same length. From , for u = 0 and constant a (acceleration due to gravity without air resistance); so s is proportional to t2, and the distance from the starting point are consecutive squares for integer values of time elapsed.[1]
The sum of the n first cubes is the square of the sum of the n first positive integers; this is Nicomachus's theorem.
All fourth powers, sixth powers, eighth powers and so on are perfect squares.
A unique relationship with triangular numbers is:
Odd and even square numbers
Squares of even numbers are even, and are divisible by 4, since (2n)2 = 4n2. Squares of odd numbers are odd, and are congruent to 1 modulo 8, since (2n + 1)2 = 4n(n + 1) + 1, and n(n + 1) is always even. In other words, all odd square numbers have a remainder of 1 when divided by 8.
Every odd perfect square is a centered octagonal number. The difference between any two odd perfect squares is a multiple of 8. The difference between 1 and any higher odd perfect square always is eight times a triangular number, while the difference between 9 and any higher odd perfect square is eight times a triangular number minus eight. Since all triangular numbers have an odd factor, but no two values of 2n differ by an amount containing an odd factor, the only perfect square of the form 2n − 1 is 1, and the only perfect square of the form 2n + 1 is 9.
Special cases
- If the number is of the form m5 where m represents the preceding digits, its square is n25 where n = m(m + 1) and represents digits before 25. For example, the square of 65 can be calculated by n = 6 × (6 + 1) = 42 which makes the square equal to 4225.
- If the number is of the form m0 where m represents the preceding digits, its square is n00 where n = m2. For example, the square of 70 is 4900.
- If the number has two digits and is of the form 5m where m represents the units digit, its square is aabb where aa = 25 + m and bb = m2. For example, to calculate the square of 57, m = 7 and 25 + 7 = 32 and 72 = 49, so 572 = 3249.
- If the number ends in 5, its square will end in 5; similarly for ending in 25, 625, 0625, 90625, ... 8212890625, etc. If the number ends in 6, its square will end in 6, similarly for ending in 76, 376, 9376, 09376, ... 1787109376. For example, the square of 55376 is 3066501376, both ending in 376. (The numbers 5, 6, 25, 76, etc. are called automorphic numbers. They are sequence A003226 in the OEIS.[2])
- In base 10, the last two digits of square numbers follow a repeating pattern mirrored symmetrical around multiples of 25. In the example of 24 and 26, both 1 off from 25, 242 = 576 and 262 = 676, both ending in 76. In general, . An analogous pattern applies for the last 3 digits around multiples of 250, and so on. As a consequence, of the 100 possible last 2 digits, only 22 of them occur among square numbers (since 00 and 25 are repeated).
Notes
- ^ Olenick, Richard P.; Apostol, Tom M.; Goodstein, David L. (2008-01-14). The Mechanical Universe: Introduction to Mechanics and Heat (dalam bahasa Inggris). Cambridge University Press. hlm. 18. ISBN 978-0-521-71592-8.
- ^ Sloane, N.J.A. (ed.). "Sequence A003226 (Automorphic numbers: n^2 ends with n.)". On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
Further reading
- Conway, J. H. and Guy, R. K. The Book of Numbers. New York: Springer-Verlag, pp. 30–32, 1996. ISBN 0-387-97993-X
- Kiran Parulekar. Amazing Properties of Squares and Their Calculations. Kiran Anil Parulekar, 2012 https://books.google.com/books?id=njEtt7rfexEC
























