Pengguna:Dedhert.Jr/Uji halaman 01/29

A Kepler triangle is a right triangle formed by three squares with areas in geometric progression according to the golden ratio.

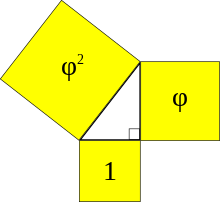
Segitiga Kepler adalah segitiga siku-siku istimewa dengan panjang sisi dalam barisan geometri. Rasio dari barisan tersebut adalah $\varphi$, yang merupakan rasio emas yang memiliki nilai $(1+{\sqrt {5}})/2$, dan barisan tersebut dapat ditulis sebagai: $1:{\sqrt {\varphi }}:\varphi$, atau sekitar $1:1,272:1,618$. Persegi-persegi dengan sisinya yang membentuk segitiga ini memiliki luas dalam barisan geometri lainnya, yaitu $1:\varphi :\varphi ^{2}$. Definisi lain dari segitiga yang sama mencirikannya dalam tiga macam rata-rata Pythagoras dari dua bilangan, atau melalui jari-jari dalam dari segitiga sama kaki.

Segitiga ini dinamai dari Johannes Kepler, tetapi segitiga ini dapat ditemukan di sumber-sumber lainnya. Walaupun beberapa sumber mengklaim bahwa piramida Mesir kuno memiliki proporsi yang didasarkan pada segitiga Kepler, banyak ahli yang percaya bahwa matematika dan arsitektur Mesir masih belum mengetahui rasio emas.

Sejarah

Segitiga Kepler dinamai dari seorang matematikawan sekaligus astronom asal Jerman yang bernama Johannes Kepler (1571–1630), yang menulis bangun tersebut dalam makalahnya tahun 1597. Dua konsep yang digunakan untuk menganalisis segitiga ini, yaitu teorema Pythagoras dan rasio emas, membuat Kepler terpesona, sehingga ia mengatakan:

Geometri memiliki dua harta yang besar. Harta yang pertama adalah teorema Pythagoras, dan harta lainnya adalah pembagian garis menjadi rasio rata-rata dan ekstrim. Kita mula-mula dapat membandingkannya dengan massa emas, dan yang kedua kita menyebutnya permata berharga.

Sayangnya, Kepler bukanlah orang yang pertama kali yang menjelaskan segitiga ini. Kepler sendiri mengaitkannya dengan "seorang guru besar musik yang bernama Magirus". Segitiga yang sama juga ditemukan di beberapa buku milik matematikawan Arab Abû Bekr, yang berjudul Liber mensurationum. Buku ini dikenal dari abad ke-12, diterjemahkan oleh Gerard of Cremona ke dalam bahasa Latin. Segitiga ini juga dapat ditemukan dalam karya Fibonacci yang berjudul Practica geometriae yang diterbitkan sekitar tahun 1220 dan 1221, dan di dalam karya tersebut mendefinisikannya dengan cara yang sama seperti Kepler. Sebelum Kepler, Pedro Nunes menulis tentang segitiga ini pada tahun 1567, dan "kemungkinan bahwa segitiga tersebut sudah terbesaar luar dalam tradisi manuskrip di abad pertengahan dan di zaman Reinsans". Sebelum Kepler juga, segitiga ini juga ditemukan kembali secara terus-menerus.

A right triangle formed by an edge midpoint, base center point, and apex of a square pyramid. Some pyramidologists have theorized that the triangle formed in this way for the Great Pyramid of Giza was intended as a Kepler triangle.

Menurut beberapa penulis, "piramid emas" dengan segitiga Kepler berganda yang dijadikan sebagai penampang lintasannya menggambarkan desain piramida Mesir seperti Piramida Agung Gaza; one source of this theory is a 19th-century misreading of Herodotus by pyramidologist John Taylor. Many other theories of proportion have been proposed for the same pyramid, unrelated to the Kepler triangle. Because these different theories are very similar in the numeric values they obtain, and because of inaccuracies in measurement, in part caused by the destruction of the outer surface of the pyramid, such theories are difficult to resolve based purely on physical evidence. The match in proportions to the Kepler triangle may well be a numerical coincidence: according to scholars who have investigated this relationship, the ancient Egyptians most likely did not know about or use the golden ratio in their mathematics or architecture. Instead, the proportions of the pyramid can be adequately explained using integer ratios, based on a right triangle with sides 11 and 14.

The name "Kepler triangle" for this shape was used by Roger Herz-Fischler, based on Kepler's 1597 letter, as early as 1979. Another name for the same triangle, used by Matila Ghyka in his 1946 book on the golden ratio, The Geometry of Art and Life, is the "triangle of Price", after pyramidologist W. A. Price.

Definisi

When an isosceles triangle is formed from two Kepler triangles, reflected across their long sides, it has the maximum possible inradius among all isosceles triangles having the same length for their two equal sides.

Segitiga Kepler didefinisikan dengan sifat-sifat yang mengharuskannya berupa segitiga siku-siku dan juga memiliki panjang sisi panjang sisinya dalam barisan geometri, atau memiliki persegi dengan sisinya dalam barisan geometri. Rasio dari barisan sisi tersebut adalah ${\sqrt {\varphi }}$, dengan $\varphi =(1+{\sqrt {5}})/2$ adalah rasio emas, dan barisan geometri tersebut dapat ditulis: $1:{\sqrt {\varphi }}:\varphi$, atau kira-kira sama dengan 1 : 1,272 : 1,618. Squares on the edges of this triangle have areas in another geometric progression, $1:\varphi :\varphi ^{2}$. The fact that the triangle with these proportions is a right triangle follows from the fact that, for squared edge lengths with these proportions, the defining polynomial of the golden ratio is the same as the formula given by the Pythagorean theorem for the squared edge lengths of a right triangle: $$\varphi ^{2}=\varphi +1.$$ Karena persamaan ini berlaku benar untuk rasio emas, ketiga panjang sisi tersebut mematuhi teorema Pythagoras, dan membentuk segitiga siku-siku. Conversely, in any right triangle whose squared edge lengths are in geometric progression with any ratio $\rho$, the Pythagorean theorem implies that this ratio obeys the identity $\rho ^{2}=\rho +1$. Therefore, the ratio must be the unique positive solution to this equation, the golden ratio, and the triangle must be a Kepler triangle.

The three edge lengths $1$, ${\sqrt {\varphi }}$ and $\varphi$ are the harmonic mean, geometric mean, and arithmetic mean, respectively, of the two numbers $\varphi \pm 1$. These three ways of combining two numbers were all studied in ancient Greek mathematics, and are called the Pythagorean means. Conversely, this can be taken as an alternative definition of the Kepler triangle: it is a right triangle whose edge lengths are the three Pythagorean means of some two numbers. The only triangles for which this is true are the Kepler triangles.

A third, equivalent way of defining this triangle comes from a problem of maximizing the inradius of isosceles triangles. Among all isosceles triangles with a fixed choice of the length of the two equal sides but with a variable base length, the one with the largest inradius is formed from two copies of the Kepler triangle, reflected across their longer sides from each other. Therefore, the Kepler triangle can be defined as the right triangle that, among all right triangles with the same hypotenuse, forms with its reflection the isosceles triangle of maximum inradius. The same reflection also forms an isosceles triangle that, for a given perimeter, contains the largest possible semicircle.

Sifat

In Coxeter's loxodromic sequence of tangent circles, the centers of each three consecutive circles form an angle from the Kepler triangle.

Jka sisi pendek dari suatu segitiga Kepler triangle memiliki panjang $s$, maka sisi lainnya memiliki panjang $s{\sqrt {\varphi }}$ dan $s\varphi$. Luas dari segitiga Kepler dapat dihitung dengan menggunakan rumus umum dari luas segitiga siku-siku, yaitu setengah dari perkalian antara dua sisi pendek, ${\tfrac {s^{2}}{2}}{\sqrt {\varphi }}$. The cosine of the larger of the two non-right angles is the ratio of the adjacent side (the shorter of the two sides) to the hypotenuse, $\varphi$, from which it follows that the two non-right angles are

$$\theta =\sin ^{-1}{\frac {1}{\varphi }}\approx 38.1727^{\circ }$$ and $$\theta =\cos ^{-1}{\frac {1}{\varphi }}\approx 51.8273^{\circ }.$$

Jerzy Kocik has observed that the larger of these two angles is also the angle formed by the centers of triples of consecutive circles in Coxeter's loxodromic sequence of tangent circles.

Lihat pula

• Automedian triangle, a triangle whose squared side lengths form an arithmetic progression, including the right triangle with side lengths $1:{\sqrt {2}}:{\sqrt {3}}$
• Golden triangle, an isosceles triangle whose ratio of base to side length is the golden ratio.